What Do Scientist Use to Determine the Likelihood of an Offspring Inheriting a Trait?

Tabular summary of genetic combinations

A Punnett square showing a typical exam cross. (green pod colour is dominant over yellowish for pea pods^[1] in dissimilarity to pea seeds, where yellow cotyledon color is dominant over dark-green^[2]).

Punnett squares for each combination of parents' colour vision status giving probabilities of their offsprings' condition, each jail cell having 25% probability in theory.

The Punnett square is a square diagram that is used to predict the genotypes of a particular cross or convenance experiment. Information technology is named after Reginald C. Punnett, who devised the approach in 1905.^{[three] [4] [5] [half-dozen] [vii] [8]} The diagram is used by biologists to determine the probability of an offspring having a detail genotype. The Punnett square is a tabular summary of possible combinations of maternal alleles with paternal alleles.^[ix] These tables tin be used to examine the genotypical upshot probabilities of the offspring of a single trait (allele), or when crossing multiple traits from the parents. The Punnett foursquare is a visual representation of Mendelian inheritance. It is important to understand the terms "heterozygous", "homozygous", "double heterozygote" (or homozygote), "dominant allele" and "recessive allele" when using the Punnett square method. For multiple traits, using the "forked-line method" is typically much easier than the Punnett foursquare. Phenotypes may exist predicted with at least amend-than-chance accuracy using a Punnett square, but the phenotype that may appear in the presence of a given genotype can in some instances be influenced past many other factors, every bit when polygenic inheritance and/or epigenetics are at work.

Zygosity [edit]

Zygosity refers to the grade of similarity betwixt the alleles that determine one specific trait in an organism. In its simplest class, a pair of alleles can exist either homozygous or heterozygous. Homozygosity, with man relating to aforementioned while zygous pertains to a zygote, is seen when a combination of either two ascendant or two recessive alleles code for the aforementioned trait. Recessive are ever lowercase messages. For case, using 'A' as the representative character for each allele, a homozygous dominant pair's genotype would be depicted equally 'AA', while homozygous recessive is shown as 'aa'. Heterozygosity, with hetero associated with unlike, can merely be 'Aa' (the uppercase alphabetic character is ever presented outset by convention). The phenotype of a homozygous dominant pair is 'A', or dominant, while the opposite is truthful for homozygous recessive. Heterozygous pairs e'er have a ascendant phenotype.^[10] To a lesser degree, hemizygosity^[11] and nullizygosity^[12] can also be seen in

Monohybrid cross [edit]

"Mono-" ways "one"; this cantankerous indicates that the test of a single trait. This could mean (for example) eye colour. Each genetic locus is e'er represented past two letters. Then in the case of eye color, say "B = Dark-brown eyes" and "b = green eyes". In this example, both parents accept the genotype Bb. For the instance of centre color, this would mean they both take dark-brown eyes. They tin can produce gametes that contain either the B or the b allele. (Information technology is conventional in genetics to use majuscule messages to indicate ascendant alleles and lower-case letters to indicate recessive alleles.) The probability of an individual offspring'southward having the genotype BB is 25%, Bb is 50%, and bb is 25%. The ratio of the phenotypes is iii:one, typical for a monohybrid cross. When assessing phenotype from this, "3" of the offspring have "Chocolate-brown" eyes and just one offspring has "green" eyes. (three are "B?" and 1 is "bb")

Paternal Maternal	B	b
B	BB	Bb
b	Bb	bb

The in which the B and b alleles interact with each other to affect the appearance of the offspring depends on how the gene products (proteins) interact (see Mendelian inheritance). This can include lethal furnishings and epistasis (where one allele masks some other, regardless of dominant or recessive status).

Dihybrid cantankerous [edit]

More than complicated crosses can exist made by looking at two or more genes. The Punnett square works, however, only if the genes are independent of each other, which ways that having a particular allele of cistron "A" does not alter the probability of possessing an allele of gene "B". This is equivalent to stating that the genes are not linked, so that the 2 genes practise not tend to sort together during meiosis.

The following example illustrates a dihybrid cross between 2 double-heterozygote pea plants. R represents the dominant allele for shape (round), while r represents the recessive allele (wrinkled). A represents the dominant allele for color (yellow), while a represents the recessive allele (dark-green). If each plant has the genotype RrAa, and since the alleles for shape and color genes are independent, then they tin produce four types of gametes with all possible combinations: RA, Ra, rA, and ra.

	RA	Ra	rA	ra
RA	RRAA	RRAa	RrAA	RrAa
Ra	RRAa	RRaa	RrAa	Rraa
rA	RrAA	RrAa	rrAA	rrAa
ra	RrAa	Rraa	rrAa	rraa

Since ascendant traits mask recessive traits (assuming no epistasis), in that location are nine combinations that have the phenotype round yellow, three that are round green, iii that are wrinkled yellowish, and 1 that is wrinkled green. The ratio 9:3:iii:1 is the expected outcome when crossing 2 double-heterozygous parents with unlinked genes. Whatever other ratio indicates that something else has occurred (such equally lethal alleles, epistasis, linked genes...etc.;).

Forked-line method [edit]

The forked-line method (also known every bit the tree method and the branching organization) can also solve dihybrid and multi-hybrid crosses. A problem is converted to a serial of monohybrid crosses, and the results are combined in a tree. Notwithstanding, a tree produces the same upshot as a Punnett foursquare in less fourth dimension and with more clarity. The example below assesses some other double-heterozygote cross using RrYy x RrYy. As stated higher up, the phenotypic ratio is expected to exist 9:3:3:ane if crossing unlinked genes from 2 double-heterozygotes. The genotypic ratio was obtained in the diagram below, this diagram will accept more branches than if only analyzing for phenotypic ratio.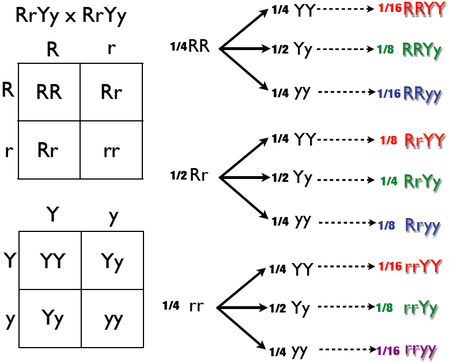

There are too Punnett squares for epistasis. In these cases the genotype epistatic over the other genes hinders their expression in the phenotype.

See also [edit]

• Mendelian inheritance
• Karnaugh map, a like diagram used for Boolean algebra simplification

References [edit]

1. ^ Mendel, Gregor Johann (1866) [1865]. Versuche über Pflanzen-Hybriden. BSHS Translations. Verhandlungen des naturforschenden Vereins (in High german and English). Vol. IV (Separate ed.). Brno: Verlag des naturforschender Vereins zu Brünn / Georg Gastl's Buchdruckerei /. p. 14. Archived from the original on 2021-03-29. Retrieved 2020-06-01 .
2. ^ Mendel, Gregor Johann (1866) [1865]. Versuche über Pflanzen-Hybriden. BSHS Translations. Verhandlungen des naturforschenden Vereins (in German and English). Vol. IV (Separate ed.). Brno: Verlag des naturforschender Vereins zu Brünn / Georg Gastl's Buchdruckerei. p. 47. Archived from the original on 2021-03-29. Retrieved 2020-06-01 .
3. ^ Punnett, Reginald Crundall (1907). Mendelism (2 ed.). London, Britain: Macmillan. (NB. The 1905 starting time edition of this volume does not contain the Punnett foursquare. In 1911, the 3rd edition gives a more than thorough caption.)
4. ^ Edwards, Anthony William Fairbank (March 2012). "Punnett's square". Studies in History and Philosophy of Science Part C: Studies in History and Philosophy of Biological and Biomedical Sciences. 43 (ane): 219–224. doi:ten.1016/j.shpsc.2011.11.011. PMID 22326091. Abstract: The origin and development of Punnett's Square for the enumeration and display of genotypes arising in a cross in Mendelian genetics is described. Due to R. C. Punnett, the idea evolved through the work of the 'Cambridge geneticists', including Punnett'southward colleagues William Bateson, E. R. Saunders and R. H. Lock, soon after the rediscovery of Mendel'southward paper in 1900. These geneticists were thoroughly familiar with Mendel's paper, which itself independent a similar square diagram. A previously-unpublished three-factor diagram past Sir Francis Galton existing in the Bateson correspondence in Cambridge Academy Library is and then described. Finally the connectedness between Punnett's Square and Venn Diagrams is emphasized, and it is pointed out that Punnett, Lock and John Venn overlapped as Fellows of Gonville and Caius College, Cambridge.
5. ^ Edwards, Anthony William Fairbank (September 2012). "Reginald Crundall Punnett: First Arthur Balfour Professor of Genetics, Cambridge, 1912". Perspectives. Genetics. Gonville and Caius College, Cambridge, United kingdom: Genetics Social club of America. 192 (1): three–13. doi:ten.1534/genetics.112.143552. PMC3430543. PMID 22964834. pp. 5–vi: […] Punnett's square seems to have been a evolution of 1905, too late for the showtime edition of his Mendelism (May 1905) but much in evidence in Report III to the Evolution Commission of the Imperial Society [(Bateson et al. 1906b) "received March 16, 1906"]. The earliest mention is contained in a letter to Bateson from Francis Galton dated October ane, 1905 (Edwards 2012). We have the testimony of Bateson (1909, p. 57) that "For the introduction of this system [the 'graphic method'], which greatly simplifies difficult cases, I am indebted to Mr. Punnett." […] The commencement published diagrams appeared in 1906. […] when Punnett published the second edition of his Mendelism, he used a slightly different format ([…] Punnett 1907, p. 45) […] In the tertiary edition (Punnett 1911, p. 34) he reverted to the organisation […] with a description of the construction of what he called the "chessboard" method (although in truth it is more similar a multiplication tabular array). […] (11 pages)
6. ^ Wimsatt, William C. (2012-05-15), "The analytic geometry of genetics: Office I: the structure, function, and early evolution of Punnett squares", Annal for History of Exact Sciences, 66 (66): 359–396 [359], doi:x.1007/s00407-012-0096-seven, S2CID 119557681
7. ^ Edwards, Anthony William Fairbank (June 2016). "Punnett's square: a postscript". Studies in History and Philosophy of Science Part C: Studies in History and Philosophy of Biological and Biomedical Sciences. Elsevier Ltd. 57: 69–lxx. doi:x.1016/j.shpsc.2016.01.001. Retrieved 2021-03-29 . (2 pages)
8. ^ Müller-Wille, Staffan; Parolini, Giuditta (2020-12-09). "Punnett squares and hybrid crosses: how Mendelians learned their trade by the book". Learning by the Volume: Manuals and Handbooks in the History of Scientific discipline. BJHS Themes. Vol. 5. British Society for the History of Science / Cambridge University Printing. pp. 149–165. doi:10.1017/bjt.2020.12. S2CID 229344415. Archived from the original on 2021-03-29. Retrieved 2021-03-29 . […] Nilsson-Ehle was experimenting with a visual arrangement that would become very popular in Mendelian genetics. The lower half of his notes comes close to what is known every bit the 'Punnett square' […] Punnett introduced this square diagram to the literature in 1906 in a paper co-authored with Bateson and Edith R. Saunders, and included it in the second edition of his Mendelism. In the third edition (1911), he added a verbal description of how to construct the diagram, and the Punnett square became a standard feature of Mendelian literature. Every bit a detailed reconstruction past A.W.F. Edwards has shown, the diagram first took shape in an exchange of letters between Bateson and Galton for the more than complex case of a trihybrid cross, and may well take been inspired by the mode in which Mendel presented a case of trifactorial inheritance of bloom colour in beans. […]
9. ^ Griffiths, Anthony J. F.; Miller, Jeffrey H.; Suzuki, David T.; Lewontin, Richard C.; Gelbart, William M. (2000). An Introduction to Genetic Assay (7 ed.). New York, Us: W. H. Freeman.
10. ^ AthenaMyth (2014-06-16). "Dominant/Recessive vs Hetero/Homozygous". DeviantArt. Archived from the original on 2021-03-29. Retrieved 2017-xi-19 .
11. ^ Shiel Jr., William C. (2018-12-12) [2017]. "Medical Definition of Hemizygous". MedicineNet. MedicineNet, Inc. Archived from the original on 2021-03-29. Retrieved 2017-11-19 .
12. ^ Robles, Ivan Suarez (2010-eleven-16). "nullizygous". Huntington's Outreach Project for Education, at Stanford (hopes). spider web.stanford.edu. Archived from the original on 2021-03-29. Retrieved 2017-11-19 .

Further reading [edit]

• Campbell, Neil Allison (2005). Biology (7 ed.). Benjamin-Cummings Publishing Company. ISBN978-0-8053-7146-8. OCLC 71890442.

External links [edit]

• Online Punnett Foursquare Reckoner
• Online Punnett Square Computer, monohybrid and dihybrid, autosomal and sex-linked

grahamancomettiody.blogspot.com

Source: https://en.wikipedia.org/wiki/Punnett_square

Share this post